1.5 Calculation of Radiometric Quantities (Examples)
Example 1: Isotropic point source
A small source emits light equally in all directions (spherical symmetry). Its radiant power equals Φe,source = 10 W.
If we are interested in the characteristics of this source at a distance (r) that is much larger than the geometric dimensions of the source itself, we can neglect the actual size of the source and assume that the light is emitted from a point. As a rule of thumb, this approximation is justified if distance r is at least 10 times larger than the dimensions of the light source.
a) Since the source emits light symmetrical in all directions, its radiant intensity is equal for all directions and amounts to
Ie = Φe,source = 10 W = 0.796 W / sr 4π sr 4π sr
b) An infinitesimal surface element dA at a distance r and perpendicular to the beam occupies the solid angle
dΩ = dA r²
and thus the infinitesimal radiant power dΦe,imp impinging onto dA can be calculated by
dΦe,imp = I dΩ = Φe,source × dA = Φe,source × dA 4π sr r2 4π r2
The irradiance at distance r therefore amounts to
Ee = Φe,source 4π r²
This result can also be obtained by the following argument:
At distance r, all the radiant power Φe,source emitted by the source passes through the surface of a sphere with radius r, which is given by 4r²π. Because the light source emits light symmetrically in all directions, the irradiance has the same value at every point of this sphere. Thus, irradiance E of a surface at a certain distance r and oriented perpendicular to the beam can be calculated from its definition:
Ee = radiant power impinging upon a surface or area of this surface = Φe,source 4π r²
which is identical with the result above.
Remark: The proportionality of E to r² is generally described with the “inverse square law”. However, it only holds true for distances much larger than the geometric dimensions of the source, which allows the assumption of a point source. In other cases, a source with considerable geometric dimensions might possibly be replaced by a “virtual” point source, for which the “inverse square law” would still apply at a distance r from this virtual point source (see Example 2). However, when the source cannot be equated with a point source and every point of the source emits light in more than a single direction, the “inverse square law” can no longer be applied. Fluorescent tubes are a good example in this case.
Example 2: Spot source
In a simple flashlight, a concave mirror reflects light from a small bulb (radiant power Φ = 200 mW) into a divergent cone (see Fig. 1). Assuming that the mirror reflects without any losses a uniform distribution of power over the cone is generated.
Note that the flashlight does not emit light symmetrically in all directions and the equations derived in Example 1 can therefore not be used.
a) At a distance of 25 cm from the flashlight’s front window, the whole radiant power of 200 mW (= 0.2 W) impinges on a circle with a 0.05 m radius. If we assume that irradiance is constant all over this circle and we neglect the fact that the surface is not perfectly even hence not strictly perpendicular to the beam, we can calculate the irradiance at a 25 cm distance from the flashlight’s front window:
E = radiant power impinging upon a surface or area of this surface = 0.2 W / m² 0.052 π E ≈ 25 W / m²
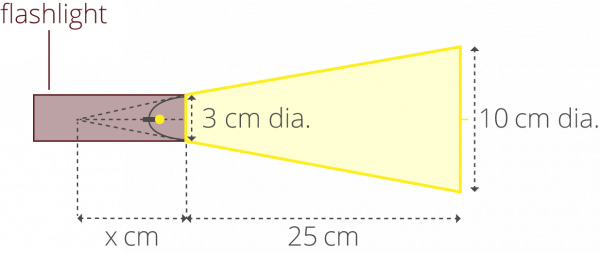
Fig. 1: Calculating the irradiance caused by a flashlight
Source (valid as of 2002): Based on http://omlc.ogi.edu/classroom/ece532/class1/intensity_flashlight.html
b) In order to determine the flashlight’s radiant intensity, we have to determine the solid angle determined by the cone. Following the definition of solid angle and approximating the area of the spherical calotte using the area of a circle with a 5 cm radius (= 0.05 m), we get
Ω = Acircle r²
where r is the distance of the circle from the cone’s vertex.
From Fig. 1, we get
r = x + 0.25 m
and
x = 0.03 x + 0.25 0.10
which in turn gives
x = 0.107 m
and
r = 0.357 m
Thus, the cone defines a solid angle given by
Ω = Acircle = 0.05² π = 0.0616 sr r² 0.3572
and the flashlight’s radiant intensity amounts to
I = Φ = 0.2 W = 3.25 W / sr Ω 0.616 sr
Remark: Since a virtual point source located at the cone’s vertex produces the same spatial radiation distribution as the flashlight’s bulb together with its concave mirror, the “inverse square law” applies for this configuration. However, the distance r which the law relates to has to be measured from the position of the virtual point source.
Example 3: The Lambertian surface
By definition, a Lambertian surface either emits or reflects radiation with constant radiance (Le) in all directions of a hemisphere (s. Fig. 2). Equation 2 shows that the directional distribution of radiant intensity is given by
Ie(ϑ) = Ie,0 × cos(ϑ)
with
Ie,0 = ∫ Le cos(0) dA = ∫ Le dA emitting surface emitting surface
where Ie,0 denotes radiant intensity emitted in the direction perpendicular to the surface and Ie(ϑ) denotes radiant intensity emitted in a direction enclosing the angle ϑ with the surface’s normal. Calculating the surface’s exitance Me from Equation 4 using the relation dΩ = sin(ϑ) dϑ dφ gives:
Me = ∫ Le cos(ϑ) dΩ = Le 2π ∫ π/2 ∫ cos(ϑ) sin(ϑ) dϑ dφ = Le × π 2πsr 0 0
The respective relations for photometric quantities (see Basic photometric quantities) characterizing a Lambertian surface can be derived by replacing the index “e” with the index “v”.
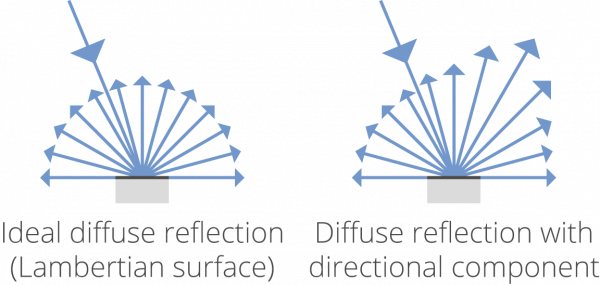
Fig. 2: Constant spatial distribution of radiance Le after ideal diffuse reflection on a Lambertian surface
Reflecting Lambertian surfaces are widely used in light measurement for well defined, perfectly diffuse scattering that is fully independent of the direction of incoming beams. Thus, the radiance reflected in a certain direction from a certain location on the surface is proportional to the total radiant power impinging onto the reflecting surface. This allows the realization of detector geometries for radiant power, exitance and irradiance (or luminous flux, luminous exitance and illuminance), which have to be determined through integration over all directions of a solid angle of 4π or 2π. Lambertian reflection is of particular interest in the coating of integrating spheres, which are widely used for detector input optics or output optics of radiance or luminance standards.